Implementation
At Rosehill Infant School, the teaching of mathematics is underpinned by the following aims:
- To develop conceptual understanding by using models, pictorials and concrete resources so that children understand the mathematics that they are learning and are not just taught ‘tricks’.
- To highlight and utilize relationships between concepts and procedures.
- To encourage mathematical reasoning by following lines of enquiry, generalising and justifying using mathematical language.
- To apply mathematical understanding to problem solving by breaking down problems into simpler steps and persevering in seeking solutions using a range of strategies.
- To develop resilient children who are confident and enthused about mathematics
- To provide ‘purposeful maths’ through application of mathematical skills and knowledge to the world around them.
How Mathematical skills are taught
Mathematical skills are taught using concrete, pictorial and abstract methods.
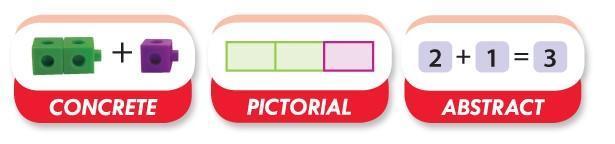
Concrete
Children first learn skills using concrete objects to demonstrate their understanding. This is done through using a range of concrete resources represented in different ways linked to the learning objective.
Pictorial
After learning skills using concrete objects, children learn pictorial methods of representing their work to show their thinking processes. Pictorial is the “seeing” stage, using representations of the objects to model problems. This stage encourages children to make a mental connection between the physical object and abstract levels of understanding by drawing or looking at pictures, circles, diagrams or models which represent the objects in the problem.
Abstract
Only once a child has demonstrated that they have a solid understanding of the “concrete” and “pictorial” representations of the problem, the children are introduced to the more “abstract” concept, such as mathematical symbols. Children are introduced to the concept at a symbolic level, using only numbers, notation, and mathematical symbols, for example +, –, x, / to indicate addition, multiplication, or division.
e.g. 6 + 1 =
9 - 5 =
20 x 2 =
How we teach Mathematics in Key Stage One at Rosehill
- Whole class together – we teach mathematics to whole classes and do not label children. Lessons are planned based on formative assessment of what students already know and we include all children in learning mathematical concepts. At the planning stage, teachers consider the scaffolding that may be required for children struggling to grasp concepts in the lesson and suitable challenge questions for those who may grasp the concepts rapidly. In line with NCETM advice, one form of depth frequently used, during the first part of the lesson, is variation theory (conceptual and procedural). Variation is one of the five ‘big ideas’ at the heart of Teaching for Mastery. For example, a child who can produce a quick correct answer may be asked to solve the question using more than one other procedure, to represent the question in more than one way (such as the bar model or part whole).
- Longer but deeper – in order to ensure children have a secure and deep understanding of the content taught, our plans have been adjusted to allow longer on topics and we move more slowly through the curriculum. We use the Power Maths planning in line with White Rose Maths and ideas from Maths no problem textbooks to support progression and variation. Teachers adapt each lesson to meet the needs of their children and add extra questioning / tasks which will allow children to learn the content more deeply. The learning will focus on one key conceptual idea and connections are made across mathematical topics. To outsiders it may appear that the pace of the lesson is slower, but progress and understanding is enhanced.
- Key learning points are identified during planning (collaboratively in year teams and where possible supported by Miss Asia) and a clear journey through the maths should be shown on flipcharts (also reflected on working walls). Learning points may appear to be very small but this is deliberate. For example, a whole lesson may be spent on adding the ones to a 2 digit number. The expectation is that every child will master the concept and some children will work more deeply on the same concept using variation theory and challenge tasks.
- Questions will probe pupil understanding throughout, taking some children’s learning deeper. Responses are expected in full sentences, using precise mathematical vocabulary and stem sentences.
- Fluency – there is a whole school focus on developing an instant recall of key facts, such as number bonds, times tables, division facts, addition and subtraction facts. This will be seen in maths lessons and during Fast Maths.
